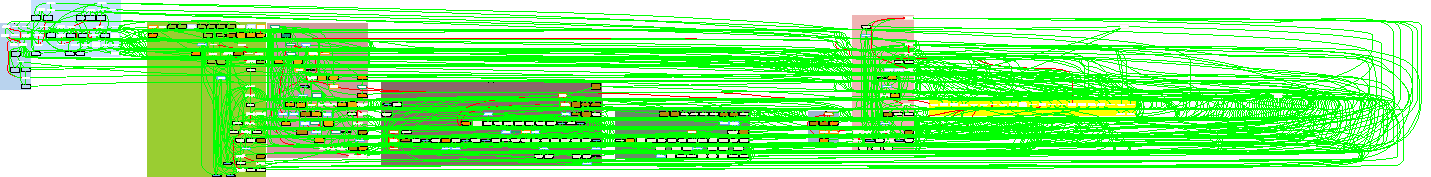
Creative Topics
We have two creative topics that are judged independently:
- Movie Remakes: This graph models remakes of movies by different directors
- Argumentation Network: shows a logical reconstruction of a scientific debate among 19th century geologists, namely the Great Devonian Controversy.
Participants may submit drawings of both graphs (or only one graph).
Movie Remakes
A movie remake is a production of a film that is based upon an earlier production. A remake tells the same story as the original but uses a different cast and may alter the theme or target audience. See [1] for related work on this topic.
For this topic, you have the task to visualize a graph of movie remakes by different directors. The data contains a list of directors, and pairs of movies: the original and the remake (both with title, year, and directors). The data has been crawled from Wikipedia and consists of 91 directors and 102 pairs of movies. You are free to decide which parts of the data to visualize and how to visualize it. The data can be downloaded here.
Spreadsheet remakes_nodes lists the directors:
| id | the id of the directors |
| name | the name of the director |
Spreadsheet remakes_edges lists the pairs of movies with the original and the remake:
| director_original | the id of the director of the original movie |
| director_remake | the id of the director of the remake |
| title_original | the title of the original movie |
| year_original | the year that the original movie was released |
| title_remake | the title of the remake |
| year_remake | the year that the remake was released |
[1] Tang Y, Yu J, Li C, Fan J. Visual Analysis of Multimodal Movie Network Data Based on the Double-Layered View. International Journal of Distributed Sensor Networks. October 2015. doi: 10.1155/2015/906316
Argumentation Network: The Great Devonian Controversy – A Logical Reconstruction
The network shows a logical reconstruction of a scientific debate among 19th century geologists, namely the Great Devonian Controversy. The network is available here.
The network contains 335 vertices which are of two types: statements and arguments (“statement-map-node” or “argument-map-node” specified in column C). Each argument has one or more sentences as premises and one sentence as a conclusion.
The vertices are given in the 12 tabs corresponding to the thematic clusters:
- Evidence
- Fossils in Pre-Old Red Sandstone Rocks
- Fossils and Time
- Dating of the Main Culm
- Dating of the Non-Culm
- Dating of the Culm Limestone
- Old Red Sandstone Characteristics
- Youngest Devonian Strata
- Gap in the Sequence of Devonshire
- Other Regions Than Devonshire
- Universalities (this is not really a cluster but two stand-alone nodes not belonging to any other cluster)
Each vertex has two labels: the short one (column B) and the full one (column C), which sometimes coincide.
The network contains 1016 edges. The edges connect statements to statements and statements to arguments. All edges are given in the tab “Edges”. Each edge has an associated type (column C). The edge types are described in the tab “Description of Edge Types” and are as follows.
The edges of type entails, contrary and contradictory are possible among the sentence vertices. An edge (p,q), where p,q are statements is interpreted as follows:
| contrary | Sentence p and q are contrary | It is not possible to accept p and q at the same time |
| contradictory | Sentence p and q contradictory | It is neither possible to accept nor to reject p and q at the same time |
| entails | Sentence p entails sentence q | It is not possible to to accept p and reject q at the same time |
The edge types attack and support are possible between a sentence and an argument in both directions. Thus edges (p,A) and (A,p) where p is a statement and A is an argument are interpreted as follows.
| (A,p) | ||
| attacks | Argument A attacks sentence p | The conclusion of A is the negation of p |
| supports | Argument A supports sentence p | The conclusion of A is p |
| (p,A) | ||
| attacks | Sentence p attacks argument A | The negation of p figures as a premise of A |
| supports | Sentence p supports argument A | p is a premise of A |
Background Information
The Great Devonian controversy spans less than a decade, from 1834 to 1840. In the beginning, opinions seem to be divided over a single question, namely whether the Main Culm strata in north-west Devon are Cambrian or Coal Measures in age. Taking a closer look, it becomes clear that there are whole belief systems at stake, even at the very beginning. Not only dating hypotheses are debated, but also observational claims, dating principles and other auxiliary assumptions as for example the existence of local variations in fauna and flora at all times or the characteristic flora and fauna of a certain time. Even at the very beginning, the controversy takes place in a global context. All over Europe and North America, scientists participate providing new observations indirectly attacking or supporting some dating principle or auxiliary assumption. As the controversy evolves, the status of a very British formation, the Old Red Sandstone, as general type for its epoch gets more and more questionable. The substitution of 'Old Red Sandstone' by 'Devonian' is proposed - despite these two formations being very unsimilar, both in fossils and rock type. By the end of the controversy, not least because of Russian observations, ‘Devonian’ is used to term all the great intermediate deposits between the Silurian and Carboniferous system, and is applied to rocks of every variety of mineral structure providing the characteristic fossil species assemblage.
Why is this controversy great? There are several reasons. First, starting as a disagreement confined to the interpretation of plant fossils in the Culm strata of north-west Devon, the controversy ends with the establishment of a new geological period, the Devonian. Second, starting with two parties facing each other rather irreconcilable, the controversy ends with a consensus between all the most active and important geologists of that time. Third, the controversy exhibits an unrivaled richness of documentation. For all these reasons, the Great Devonian Controversy offers great insights into the shaping of scientific knowledge and should be worthwhile further attention!
The reconstruction relies on [2] and makes use of [1] and [3].
Bibliography
[1] Gregor Betz. Theorie dialektischer Strukturen. Klostermann, Frankfurt am Main, 2010.
[2] M. J. S Rudwick. The great Devonian controversy the shaping of scientific knowledge among gentlemanly specialists. University of Chicago Press, Chicago, 1988.
[3] Christian Voigt. Argdown - A Simple Syntax for Complex Argumentation, https://argdown.org, 2018.
Evaluation
For the creative topics, you are completely free to use any drawing style you wish. Your submissions will be judged on a list of criteria that includes, but is not limited to, readability, aesthetics, novelty, and design quality. The weighting of the criteria might be different for the two graphs.
Submission
Submissions will be handled through EasyChair at the web site
and must be received by September 08, 2021. The submission must be a pdf version of your visualization, suitable for printing on a large A0 poster. Your submission should also include the following information, either added to the pdf file, or in the abstract:
- A brief description on how the graph and layout were produced.
- (Optional) A link to a visualization illustrating the graph in a format of your choosing (e.g., an interactive tool).
- (Optional) A link to a web-friendly image of your visualization, max resolution 1000 by 1000 pixels. If not provided, we will use a picture of the submitted poster for the website.
If your drawing requires special printing because of size, resolution, or color constraints, you are encouraged to submit via hard-copy to the address below. In this case, please contact us in advance and make sure that your submission is received no later than September 05. Please still submit the remaining items via EasyChair.
Philipp Kindermann
Universität Trier
Fachbereich IV - Informatikwissenschaften
Lehrstuhl für Algorithmik
Campus II - Gebäude H - Raum 427
54286 Trier
Germany